This post covers Wireless Communications: Principles and Practices by Theodore S. Rapport.

Basic Ideas

• The Cellular Concept- Fundamental System Design

• Interferences

  • Susceptibility and interference problems associated with mobile communications equipment are because of the problem of time congestion within the electromagnetic spectrum.
  • It is the limiting factor in the performance of cellular systems.
  • This interference can occur from clash with another mobile in the same cell or because of a call in the adjacent cell.
  • There can be interference between the base stations operating at same frequency band or any other non-cellular system’s energy leaking inadvertently into the frequency band of the cellular system.
  • If there is an interference in the voice channels, cross talk is heard will appear as noise between the users.
  • The interference in the control channels leads to missed and error calls because of digital signaling. Interference is more severe in urban areas because of the greater RF noise and greater density of mobiles and base stations.
  • The interference can be divided into 2 parts: co-channel interference and adjacent channel interference.

• Co-channel interference (CCI)

  • For the efficient use of available spectrum, it is necessary to reuse frequency bandwidth over relatively small geographical areas.

  • However, increasing frequency reuse also increases interference, which decreases system capacity and service quality.

  • The cells where the same set of frequencies is used are call co-channel cells.

  • Co-channel interference is the cross talk between two different radio transmitters using the same radio frequency as is the case with the co-channel cells.

  • The reasons of CCI can be because of either adverse weather conditions or poor frequency planning or overly crowded radio spectrum.

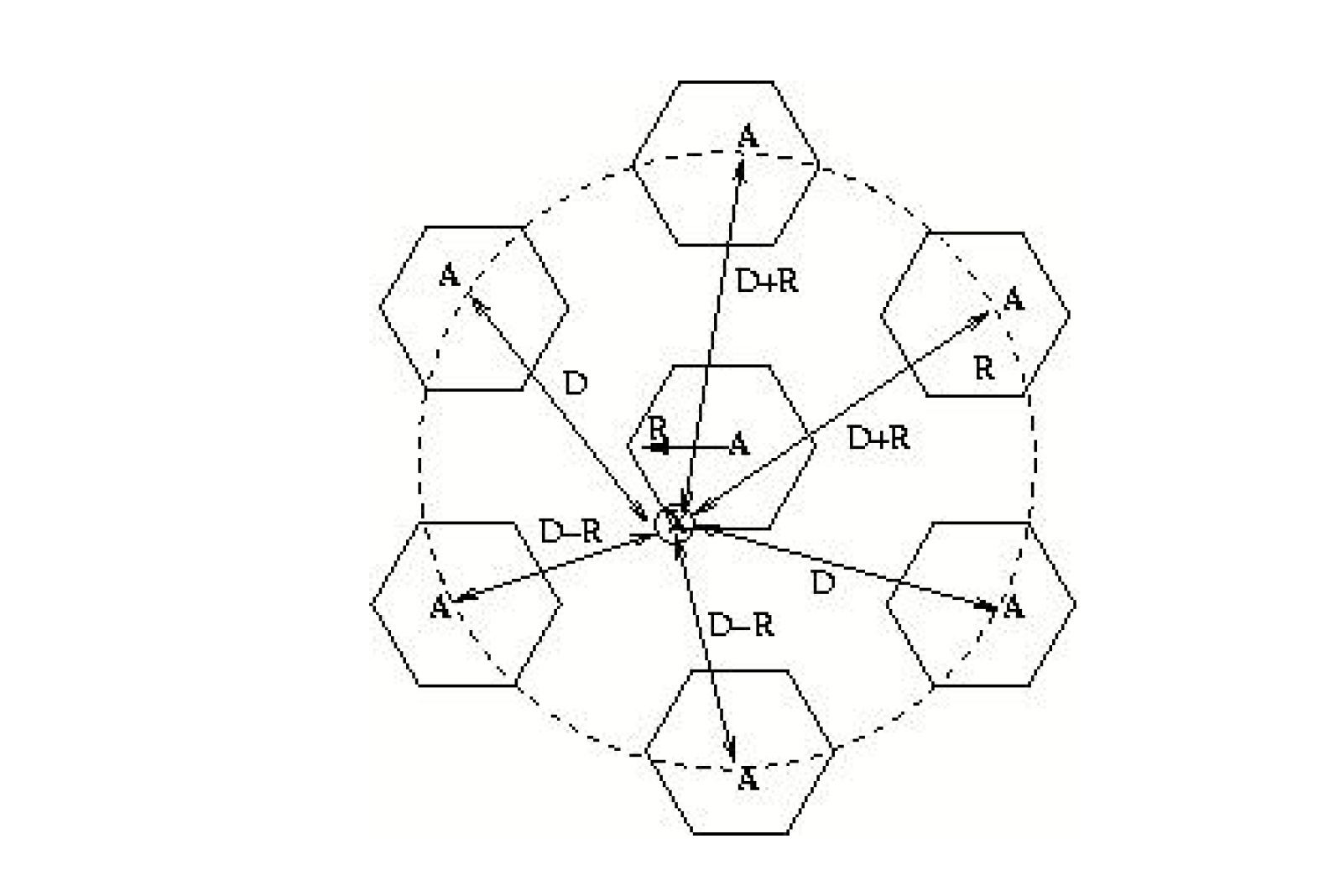
  • If the cell size and the power transmitted at the base stations are same then CCI will become independent of the transmitted power and will depend on radius of the cell (R) and the distance between the interfering co-channel cells (D).

  • If $D/R$​​ ratio is increased, then the effective distance between the co-channel cells will increase and interference will decrease. The parameter Q is called the frequency reuse ratio and is related to the cluster size. For hexagonal geometry \(Q = \frac {D}{R} = \sqrt{3N}\\\)

  • From the above equation, Q means small value of cluster size N and increase in cellular capacity and increase in cellular capacity and increase in cellular capacity.

  • But large Q leads to decrease in system capacity but increase in transmission quality. Choosing the options is very careful for the selection of N, the proof of which is given in the first section.

  • The Signal to Interference Ratio (SIR) for a mobile receiver which monitors the forward channel can be calculated as \(\frac{S}{I}= \frac{S}{\Sigma_{i=1}^{i_0}I_{i}}\)

  • where $i_0$ is the number of co-channel interfering cells, S is the desired signal power from the baseband station and Ii is the interference power caused by the $i^{th}$ interfering co-channel base station.

  • In order to solve this equation from power calculations, we need to look into the signal power characteristics.

  • The average power in the mobile radio channel decays as a power law of the distance of separation between transmitter and receiver.

  • The expression for the received power $Pr$ at a distance d can be approximately calculated as \(P_r = P_0(\frac{d}{d_0})^{-n}\) and in the dB expression as \(P_r(dB) = P_0(dB)-10nlog\frac{d}{d_0}\)

  • where $P_0$ is the power received at a close-in reference point in the far eld region at a small distance do from the transmitting antenna, and `n’ is the path loss exponent.

  • Let us calculate the SIR for this system. If $D_i$ is the distance of the i-th interferer from the mobile, the received power at a given mobile due to i-th interfering cell is proportional to ($D_i$) (the value of ‘n’ varies between 2 and 4 in urban cellular systems).

  • Let us take that the path loss exponent is same throughout the coverage area and the transmitted power be same, then SIR can be approximated as \(\frac{S} {I} = \frac{R^{-n}}{ \Sigma_{i=1}^{i_0}D^{-n}_{i}}\\\)

  • where the mobile is assumed to be located at R distance from the cell center.

  • If we consider only the first layer of interfering cells and we assume that the interfering base stations are equidistant from the reference base station and the distance between the cell centers is $’D’$ then the above equation can be converted as \(\frac{S} {I} = \frac{\frac{D}{R}^{-n}} {i_0} = ({\frac{\sqrt{3N}}{i_0}})^ {-n} \\\)

  • which is an approximate measure of the SIR. Subjective tests performed on AMPS cellular system which uses FM and 30 kHz channels show that sucient voice quality can be obtained by SIR being greater than or equal to 18 dB.

  • If we take $n=4$, the value of ‘N’ can be calculated as $6.49$. Therefore minimum N is 7.

  • The above equations are based on hexagonal geometry and the distances from the closest interfering cells can vary if different frequency reuse plans are used.

  • We can go for a more approximate calculation for co-channel SIR. This is the example of a 7 cell reuse case. \(\frac{S}{I} = \frac{R^{-4}}{2(D-R)^{-4} + (D + R)^{-4} + (D)^{-4} + (D + \frac{R}{2})^{-4} + (D - \frac{R}{2})^{-4}}\) which can be rewritten in terms frequency reuse ratio Q as \(\frac{S}{I} = \frac{1}{2(Q-1)^{-4} + (Q + 1)^{-4} + (Q)^{-4} + (Q + \frac{1}{2})^{-4} + (Q - \frac{1}{2})^{-4}}\\\)

  • The mobile is at a distance of $D-R$ from 2 closest interfering cells and approximately $D+R/2$, $D$, $D-R/2$ and $D+R$ distance from other interfering cells in the first tier.

  • Taking n = 4 in the above equation, SIR can be approximately calculated as

  • Using the value of N equal to 7 (this means Q = 4.6), the above expression yields that worst case SIR is $53.70$ ($17.3$ dB).

  • This shows that for a 7 cell reuse case the worst case SIR is slightly less than 18 dB.

  • The worst case is when the mobile is at the corner of the cell i.e., on a vertex as shown in the Figure. Therefore N = 12 cluster size should be used. But this reduces the capacity by $7/12$ times.

  ​

  • Therefore, co-channel interference controls link performance, which in a way controls frequency reuse plan and the overall capacity of the cellular system.
  • The effect of co-channel interference can be minimized by optimizing the frequency assignments of the base stations and their transmit powers.
  • Tilting the base-station antenna to limit the spread of the signals in the system can also be done.

• Adjacent Channel Interference (ACI)

  • This is a dierent type of interference which is caused by adjacent channels i.e.

    channels in adjacent cells.

  • It is the signal impairment which occurs to one frequency due to presence of another signal on a nearby frequency.

  • This occurs when imperfect receiver lters allow nearby frequencies to leak into the passband.

  • This problem is enhanced if the adjacent channel user is transmitting in a close range compared to the subscriber’s receiver while the receiver attempts to receive a base station on the channel. This is called near-far effect.

  • The more adjacent channels are packed into the channel block, the higher the spectral efficiency, provided that the performance degradation can be tolerated in the system link budget.

  • This effect can also occur if a mobile close to a base station transmits on a channel close to one being used by a weak mobile.

  • This problem might occur if the base station has problem in discriminating the mobile user from the “bleed over” caused by the close adjacent channel mobile.

  • Adjacent channel interference occurs more frequently in small cell clusters and heavily

    used cells.

  • If the frequency separation between the channels is kept large this interference can be reduced to some extent.

  • Thus assignment of channels is given such that they do not form a contiguous band of frequencies within a particular cell and frequency separation is maximized.

  • Efficient assignment strategies are very much important in making the interference as less as possible.

  • If the frequency factor is small then distance between the adjacent channels cannot put the interference level within tolerance limits.

  • If a mobile is 10 times close to the base station than other mobile and has energy spill out of its passband, then SIR for weak mobile is approximately \(\frac{S}{I}= 10^{-n}\)

• which can be easily found from the earlier SIR expressions.

• If n = 4, then $SIR$ is $52$ dB. Perfect base station lters are needed when close-in and distant users share the same cell.

• Practically, each base station receiver is preceded by a high Q cavity filter in order to remove adjacent channel interference.

• Power control is also very much important for the prolonging of the battery life for the subscriber unit but also reduces reverse channel SIR in the system.

• Power control is done such that each mobile transmits the lowest power required to maintain a good quality link on the reverse channel.

​